drawing traces to sketch 3d object

12.6: Quadric Surfaces

Last updated
Save as PDF

Page ID: 2591

Professor (Mathematics) at Massachusetts Found of Technology (Strang) & University of Wisconsin-Stevens Signal (Herman)
Source: OpenStax CNX

Learning Objectives

Place a cylinder every bit a type of three-dimensional surface.
Recognize the main features of ellipsoids, paraboloids, and hyperboloids.
Use traces to draw the intersections of quadric surfaces with the coordinate planes.

We have been exploring vectors and vector operations in iii-dimensional space, and nosotros have developed equations to describe lines, planes, and spheres. In this section, we use our knowledge of planes and spheres, which are examples of three-dimensional figures called surfaces, to explore a variety of other surfaces that tin exist graphed in a 3-dimensional coordinate system.

Identifying Cylinders

The beginning surface nosotros'll examine is the cylinder. Although nigh people immediately think of a hollow pipe or a soda straw when they hear the word cylinder, here we use the broad mathematical meaning of the term. Equally nosotros have seen, cylindrical surfaces don't have to be circular. A rectangular heating duct is a cylinder, every bit is a rolled-up yoga mat, the cross-department of which is a spiral shape.

In the two-dimensional coordinate plane, the equation $ x^2+y^2=9$ describes a circle centered at the origin with radius $ 3$. In three-dimensional space, this same equation represents a surface. Imagine copies of a circumvolve stacked on top of each other centered on the $z$-centrality (Figure $\PageIndex{i}$), forming a hollow tube. We tin then construct a cylinder from the set of lines parallel to the $z$-axis passing through the circle $ x^2+y^2=nine$ in the $xy$-plane, as shown in the figure. In this way, any bend in ane of the coordinate planes tin be extended to become a surface.

This figure a 3-dimensional coordinate system. It has a right circular center with the z-axis through the center. The cylinder also has points labeled on the x and y axis at (3, 0, 0) and (0, 3, 0). — Figure $\PageIndex{1}$: In 3-dimensional space, the graph of equation $ 10^2+y^2=9$ is a cylinder with radius $ 3$ centered on the $z$-axis. It continues indefinitely in the positive and negative directions.

Definition: cylinders and rulings

A set of lines parallel to a given line passing through a given curve is known equally a cylindrical surface, or cylinder . The parallel lines are chosen rulings.

From this definition, we tin see that we notwithstanding take a cylinder in three-dimensional infinite, even if the curve is not a circle. Any curve can grade a cylinder, and the rulings that compose the cylinder may exist parallel to any given line (Figure $\PageIndex{2}$).

This figure has a 3-dimensional surface that begins on the y-axis and curves upward. There is also the x and z axes labeled. — Figure $\PageIndex{two}$: In iii-dimensional space, the graph of equation $ z=x^3$ is a cylinder, or a cylindrical surface with rulings parallel to the $y$-axis.

Example $ \PageIndex{1}$: Graphing Cylindrical Surfaces

Sketch the graphs of the following cylindrical surfaces.

$ x^2+z^2=25$
$ z=2x^2−y$
$ y=\sin x$

Solution

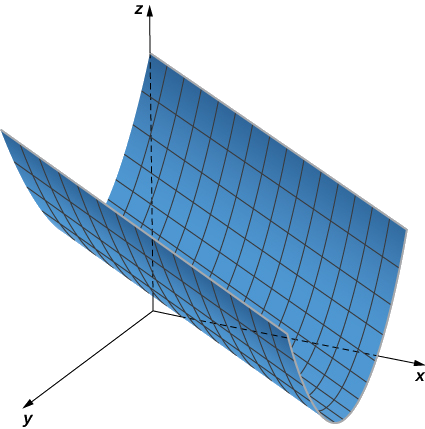
a. The variable $ y$ tin accept on any value without limit. Therefore, the lines ruling this surface are parallel to the $y$-axis. The intersection of this surface with the $xz$-plane forms a circle centered at the origin with radius $ 5$ (see Figure $\PageIndex{3}$).

This figure is the 3-dimensional coordinate system. It has a right circular cylinder on its side with the y-axis in the center. The cylinder intersects the x-axis at (5, 0, 0). It also has two points of intersection labeled on the z-axis at (0, 0, 5) and (0, 0, -5). — Effigy $\PageIndex{3}$: The graph of equation $ x^2+z^2=25$ is a cylinder with radius $ 5$ centered on the $y$-axis.

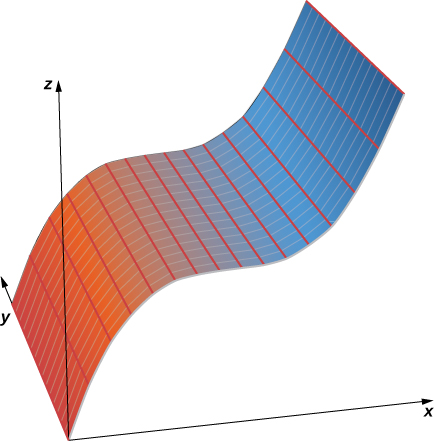
b. In this case, the equation contains all three variables —$ x,y,$ and $ z$— so none of the variables can vary arbitrarily. The easiest way to visualize this surface is to use a computer graphing utility (Effigy $\PageIndex{4}$).

This figure has a surface in the first octant. The cross section of the solid is a parabola. — Figure $\PageIndex{4}$

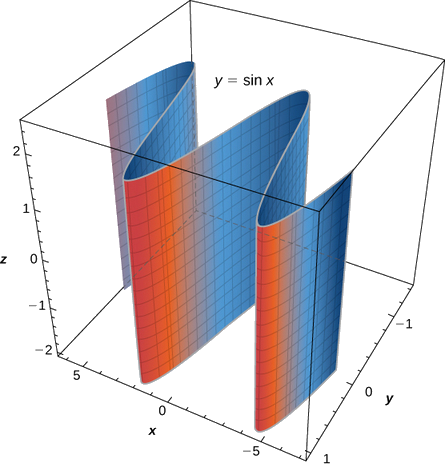
c. In this equation, the variable $ z$ tin can take on any value without limit. Therefore, the lines composing this surface are parallel to the $z$-axis. The intersection of this surface with the xy-airplane outlines curve $ y=\sin ten$ (Figure $\PageIndex{5}$).

This figure is a three dimensional surface. A cross section of the surface parallel to the x y plane would be the sine curve. — Figure $\PageIndex{v}$: The graph of equation $ y=\sin x$ is formed by a gear up of lines parallel to the $z$-axis passing through curve $ y=\sin x$ in the $xy$-airplane.

Do $ \PageIndex{1}$:

Sketch or use a graphing tool to view the graph of the cylindrical surface divers past equation $ z=y^ii$.

Hint: The variable $ x$ can take on any value without limit.
Answer: $This figure is a surface above the x y plane. A cross section of this surface parallel to the y z plane would be a parabola. The surface sits on top of the x y plane.$

When sketching surfaces, nosotros have seen that it is useful to sketch the intersection of the surface with a plane parallel to i of the coordinate planes. These curves are called traces. We tin can encounter them in the plot of the cylinder in Effigy $\PageIndex{6}$.

Definition: traces

The traces of a surface are the cross-sections created when the surface intersects a aeroplane parallel to 1 of the coordinate planes.

Traces are useful in sketching cylindrical surfaces. For a cylinder in three dimensions, though, just one set of traces is useful. Detect, in Figure $\PageIndex{vi}$, that the trace of the graph of $ z=\sin x$ in the xz-plane is useful in amalgam the graph. The trace in the xy-airplane, though, is but a series of parallel lines, and the trace in the yz-plane is simply one line.

This figure has two images. The first image is a surface. A cross section of the surface parallel to the x z plane would be a sine curve. The second image is the sine curve in the x y plane. — Figure $\PageIndex{half-dozen}$: (a) This is ane view of the graph of equation $ z=\sin x$. (b) To find the trace of the graph in the $xz$-plane, set up $ y=0$. The trace is merely a two-dimensional sine moving ridge.

Cylindrical surfaces are formed by a set of parallel lines. Non all surfaces in 3 dimensions are constructed so just, even so. We now explore more complex surfaces, and traces are an important tool in this investigation.

Quadric Surfaces

We have learned about surfaces in iii dimensions described by kickoff-order equations; these are planes. Some other common types of surfaces can be described by 2nd-order equations. We tin can view these surfaces as iii-dimensional extensions of the conic sections we discussed before: the ellipse, the parabola, and the hyperbola. We phone call these graphs quadric surfaces

Definition: Quadric surfaces and conic sections

Quadric surfaces are the graphs of equations that tin be expressed in the form

\[Ax^ii+By^two+Cz^2+Dxy+Exz+Fyz+Gx+Hy+Jz+K=0.\]

When a quadric surface intersects a coordinate aeroplane, the trace is a conic section.

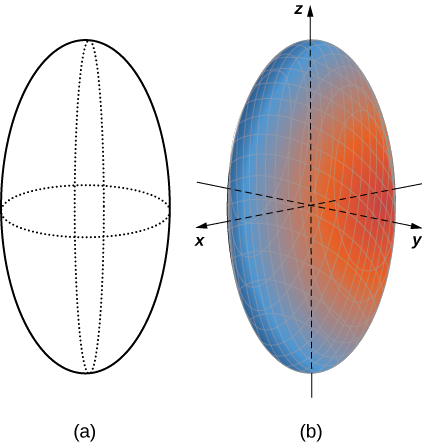
An ellipsoid is a surface described past an equation of the class $ \dfrac{x^2}{a^2}+\dfrac{y^2}{b^2}+\dfrac{z^2}{c^2}=1.$ Set up $ x=0$ to see the trace of the ellipsoid in the yz-plane. To run into the traces in the $xy$- and $xz$-planes, fix $ z=0$ and $ y=0$, respectively. Find that, if $ a=b$, the trace in the $xy$-aeroplane is a circle. Similarly, if $ a=c$, the trace in the $xz$-plane is a circle and, if $ b=c$, then the trace in the $yz$-plane is a circle. A sphere, then, is an ellipsoid with $ a=b=c.$

Example $ \PageIndex{2}$: Sketching an Ellipsoid

Sketch the ellipsoid

\[ \dfrac{ten^2}{2^two}+\dfrac{y^two}{3^2}+\dfrac{z^2}{v^two}=one.\]

Solution

Start by sketching the traces. To find the trace in the xy-plane, fix $ z=0: \dfrac{x^2}{2^2}+\dfrac{y^ii}{3^two}=1$ (Figure $\PageIndex{7}$). To find the other traces, first set up $ y=0$ and and then set $ x=0.$

This figure has three images. The first image is an oval centered around the origin of the rectangular coordinate system. It intersects the x axis at -2 and 2. It intersects the y-axis at -3 and 3. The second image is an oval centered around the origin of the rectangular coordinate system. It intersects the x-axis at -2 and 2 and the y-axis at -5 and 5. The third image is an oval centered around the origin of the rectangular coordinate system. It intersects the x-axis at -3 and 3 and the y-axis at -5 and 5. — Effigy $\PageIndex{vii}$: (a) This graph represents the trace of equation $ \dfrac{10^2}{2^2}+\dfrac{y^ii}{3^2}+\dfrac{z^ii}{v^two}=one$ in the $xy$-airplane, when nosotros set $ z=0$. (b) When we set up $ y=0$, we get the trace of the ellipsoid in the $xz$-airplane, which is an ellipse. (c) When we set $ 10=0$, nosotros go the trace of the ellipsoid in the $yz$-plane, which is likewise an ellipse.

At present that we know what traces of this solid look like, we can sketch the surface in iii dimensions (Figure $\PageIndex{eight}$).

This figure has two images. The first image is a vertical ellipse. There two curves drawn with dashed lines around the center horizontally and vertically to give the image a 3-dimensional shape. The second image is a solid elliptical shape with the center at the origin of the 3-dimensional coordinate system. — Figure $\PageIndex{8}$: (a) The traces provide a framework for the surface. (b) The centre of this ellipsoid is the origin.

The trace of an ellipsoid is an ellipse in each of the coordinate planes. Notwithstanding, this does not accept to be the case for all quadric surfaces. Many quadric surfaces have traces that are different kinds of conic sections, and this is usually indicated past the proper noun of the surface. For case, if a surface tin be described by an equation of the form

\[ \dfrac{x^ii}{a^2}+\dfrac{y^2}{b^2}=\dfrac{z}{c}\]

then we call that surface an elliptic paraboloid. The trace in the xy-aeroplane is an ellipse, just the traces in the xz-airplane and yz-plane are parabolas (Effigy $\PageIndex{9}$). Other elliptic paraboloids tin have other orientations simply by interchanging the variables to give us a dissimilar variable in the linear term of the equation $ \dfrac{x^2}{a^2}+\dfrac{z^two}{c^ii}=\dfrac{y}{b}$ or $ \dfrac{y^2}{b^2}+\dfrac{z^2}{c^two}=\dfrac{x}{a}$.

This figure is the image of a surface. It is in the 3-dimensional coordinate system on top of the origin. A cross section of this surface parallel to the x y plane would be an ellipse. — Effigy $\PageIndex{9}$: This quadric surface is called an elliptic paraboloid.

Example $ \PageIndex{3}$: Identifying Traces of Quadric Surfaces

Draw the traces of the elliptic paraboloid $ x^ii+\dfrac{y^2}{two^2}=\dfrac{z}{5}$.

Solution

To find the trace in the $xy$-plane, set $ z=0: x^2+\dfrac{y^two}{2^ii}=0.$ The trace in the aeroplane $ z=0$ is simply i point, the origin. Since a single betoken does not tell us what the shape is, we can motility up the $z$-axis to an capricious plane to detect the shape of other traces of the effigy.

The trace in plane $ z=v$ is the graph of equation $ 10^two+\dfrac{y^2}{two^two}=1$, which is an ellipse. In the $xz$-aeroplane, the equation becomes $ z=5x^2$. The trace is a parabola in this plane and in whatsoever plane with the equation $ y=b$.

In planes parallel to the $yz$-plane, the traces are also parabolas, as we can see in Figure $\PageIndex{10}$.

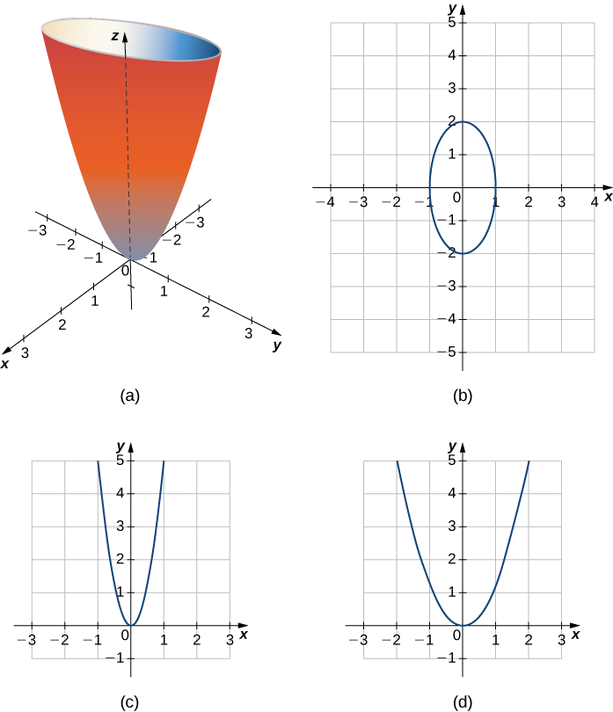
This figure has four images. The first image is the image of a surface. It is in the 3-dimensional coordinate system on top of the origin. A cross section of this surface parallel to the x y plane would be an ellipse. A cross section parallel to the x z plane would be a parabola. A cross section of the surface parallel to the y z plane would be a parabola. The second image is the cross section parallel to the x y plane and is an ellipse. The third image is the cross section parallel to the x z plane and is a parabola. The fourth image is the cross section parallel to the y z plane and is a parabola. — Figure $\PageIndex{x}$: (a) The paraboloid $ x^2+\dfrac{y^ii}{2^2}=\dfrac{z}{5}$. (b) The trace in plane $ z=v$. (c) The trace in the $xz$-plane. (d) The trace in the $yz$-plane.

Exercise $ \PageIndex{two}$:

A hyperboloid of 1 sheet is any surface that tin can be described with an equation of the grade $ \dfrac{x^2}{a^2}+\dfrac{y^2}{b^2}−\dfrac{z^2}{c^ii}=1$. Describe the traces of the hyperboloid of one canvass given past equation $ \dfrac{x^2}{three^2}+\dfrac{y^ii}{2^two}−\dfrac{z^2}{5^2}=ane.$

Hint

To find the traces in the coordinate planes, set up each variable to zero individually.

Answer

The traces parallel to the $xy$-plane are ellipses and the traces parallel to the $xz$- and $yz$-planes are hyperbolas. Specifically, the trace in the $xy$-plane is ellipse $ \dfrac{10^2}{three^ii}+\dfrac{y^two}{2^2}=1,$ the trace in the $xz$-aeroplane is hyperbola $ \dfrac{x^2}{3^2}−\dfrac{z^2}{5^2}=ane,$ and the trace in the $yz$-plane is hyperbola $ \dfrac{y^2}{2^ii}−\dfrac{z^2}{5^2}=i$ (see the following figure).

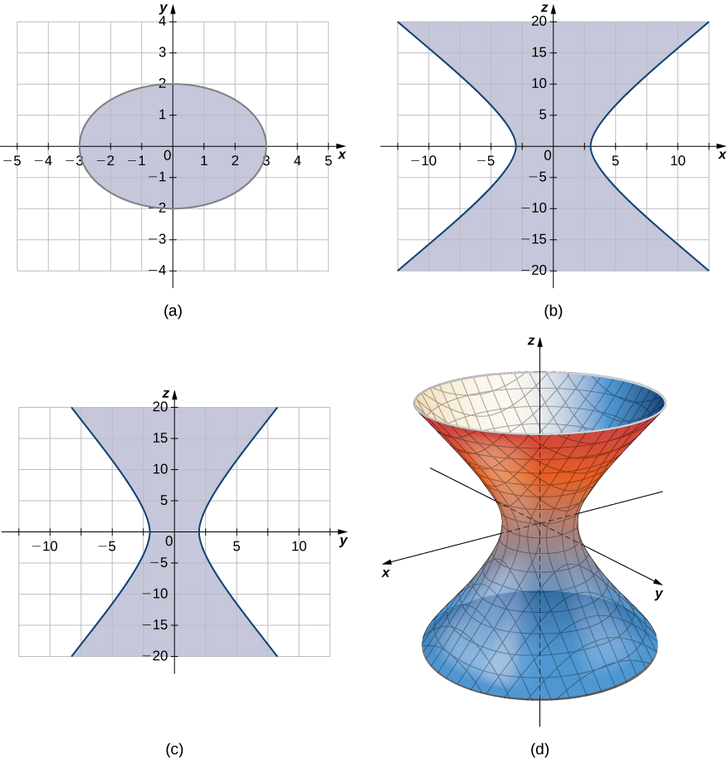
$This figure has four images. The first image is an ellipse centered at the origin of a rectangular coordinate system. It intersects the x axis at -3 and 3. It intersects the y axis at -2 and 2. The second image is the graph of a hyperbola. It is two curves one opening in the negative x direction and a symmetric one in the positive x direction. The third image is the graph of a hyperbola in the y z plane. It is opening in the negative y direction and a symmetric curve opening in the positive y direction. The fourth image is a 3-dimensional surface. It top and bottom cross sections would be circular. A vertical intersection would be a hyperbola.$

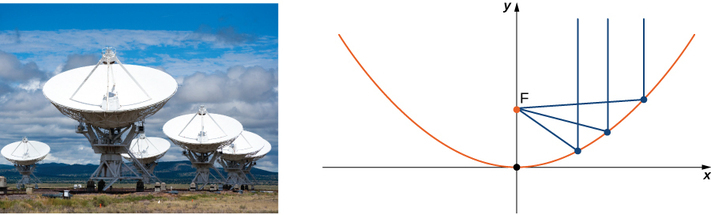
Hyperboloids of one sheet have some fascinating properties. For example, they tin can be synthetic using straight lines, such as in the sculpture in Figure $\PageIndex{1a}$. In fact, cooling towers for nuclear power plants are oft synthetic in the shape of a hyperboloid. The builders are able to utilize direct steel beams in the structure, which makes the towers very potent while using relatively little textile (Figure $\PageIndex{1b}$).

This figure has two images. The first image is a sculpture made of parallel sticks, curved together in a circle with a hyperbolic cross section. The second image is a nuclear power plant. The towers are hyperbolic shaped. — Figure $\PageIndex{11}$: (a) A sculpture in the shape of a hyperboloid can be constructed of straight lines. (b) Cooling towers for nuclear power plants are often congenital in the shape of a hyperboloid.

Example $ \PageIndex{4}$: Chapter Opener: Finding the Focus of a Parabolic Reflector

Energy hitting the surface of a parabolic reflector is concentrated at the focal indicate of the reflector (Figure $\PageIndex{12}$). If the surface of a parabolic reflector is described by equation $ \dfrac{ten^two}{100}+\dfrac{y^2}{100}=\dfrac{z}{four},$ where is the focal point of the reflector?

This figure has two images. The first image is a picture of satellite dishes with parabolic reflectors. The second image is a parabolic curve on a line segment. The bottom of the curve is at point V. There is a line segment perpendicular to the other line segment through V. There is a point on this line segment labeled F. There are 3 lines from F to the parabola, intersecting at P sub 1, P sub 2, and P sub 3. There are also three vertical lines from P sub 1 to Q sub 1, from P sub 2 to Q sub 2, and from P sub 3 to Q sub 3. — Effigy $\PageIndex{12}$: Energy reflects off of the parabolic reflector and is collected at the focal signal. (credit: modification of CGP Grey, Wikimedia Commons)

Solution

Since z is the first-power variable, the axis of the reflector corresponds to the $z$-axis. The coefficients of $ x^ii$ and $ y^two$ are equal, and then the cantankerous-section of the paraboloid perpendicular to the $z$-axis is a circumvolve. Nosotros tin consider a trace in the xz-plane or the yz-plane; the upshot is the same. Setting $ y=0$, the trace is a parabola opening upwards along the $z$-axis, with standard equation $ x^two=4pz$, where $ p$ is the focal length of the parabola. In this case, this equation becomes $ x^2=100⋅\dfrac{z}{4}=4pz$ or $ 25=4p$. And then p is $ 6.25$ m, which tells usa that the focus of the paraboloid is $ half dozen.25$ m upward the axis from the vertex. Because the vertex of this surface is the origin, the focal bespeak is $ (0,0,six.25).$

Seventeen standard quadric surfaces can exist derived from the full general equation

\[Ax^2+Past^two+Cz^2+Dxy+Exz+Fyz+Gx+Hy+Jz+1000=0.\]

The following figures summarizes the most important ones.

This figure is of a table with two columns and three rows. The three rows represent the first 6 quadric surfaces: ellipsoid, hyperboloid of one sheet, and hyperboloid of two sheets. The equations and traces are in the first column. The second column has the graphs of the surfaces. The ellipsoid graph is a vertical oblong round shape. The hyperboloid of one sheet is circular on the top and the bottom and narrow in the middle. The hyperboloid in two sheets has two parabolic domes opposite of each other. — Figure $\PageIndex{13}$: Characteristics of Common Quadratic Surfaces: Ellipsoid, **Hyperboloid of One Sheet, Hyperboloid of Two Sheets.**

This figure is of a table with two columns and three rows. The three rows represent the second 6 quadric surfaces: elliptic cone, elliptic paraboloid, and hyperbolic paraboloid. The equations and traces are in the first column. The second column has the graphs of the surfaces. The elliptic cone has two cones touching at the points. The elliptic paraboloid is similar to a cone but oblong. The hyperbolic paraboloid has a bend in the middle similar to a saddle. — Figure $\PageIndex{14}$: Characteristics of Common Quadratic Surfaces: **Elliptic Cone, Elliptic Paraboloid,** Hyperbolic Paraboloid.

Example $ \PageIndex{5}$: Identifying Equations of Quadric Surfaces

Identify the surfaces represented by the given equations.

$ 16x^2+9y^ii+16z^2=144$
$ 9x^2−18x+4y^2+16y−36z+25=0$

Solution

a. The $ 10,y,$ and $ z$ terms are all squared, and are all positive, so this is probably an ellipsoid. However, permit's put the equation into the standard grade for an ellipsoid but to be certain. We have

\[ 16x^ii+9y^2+16z^two=144. \nonumber\]

Dividing through by 144 gives

\[ \dfrac{10^ii}{9}+\dfrac{y^ii}{16}+\dfrac{z^two}{nine}=1. \nonumber\]

So, this is, in fact, an ellipsoid, centered at the origin.

b. We commencement notice that the $ z$ term is raised just to the first power, and so this is either an elliptic paraboloid or a hyperbolic paraboloid. Nosotros also annotation there are $ x$ terms and $ y$ terms that are non squared, and then this quadric surface is not centered at the origin. We need to complete the square to put this equation in one of the standard forms. We have

\[ \begin{align*} 9x^ii−18x+4y^2+16y−36z+25 =0 \\[4pt] 9x^2−18x+4y^two+16y+25 =36z \\[4pt] 9(x^2−2x)+4(y^ii+4y)+25 =36z \\[4pt] nine(ten^two−2x+ane−1)+four(y^ii+4y+four−4)+25 =36z \\[4pt] 9(x−1)^two−9+four(y+two)^two−16+25 =36z \\[4pt] ix(x−i)^2+4(y+2)^ii =36z \\[4pt] \dfrac{(x−1)^2}{iv}+\dfrac{(y−ii)^2}{9} =z. \finish{align*}\]

This is an elliptic paraboloid centered at $ (i,2,0).$

Do $ \PageIndex{3}$

Identify the surface represented by equation $ 9x^2+y^2−z^2+2z−x=0.$

Hint: Wait at the signs and powers of the $ 10,y$, and $ z$ terms
Answer: Hyperboloid of ane sheet, centered at $ (0,0,i)$.

Central Concepts

A set of lines parallel to a given line passing through a given curve is called a cylinder, or a cylindrical surface. The parallel lines are chosen rulings.
The intersection of a iii-dimensional surface and a plane is called a trace. To find the trace in the $xy$-, $yz$-, or $xz$-planes, set up $ z=0,ten=0,$ or $ y=0,$ respectively.
Quadric surfaces are three-dimensional surfaces with traces composed of conic sections. Every quadric surface tin be expressed with an equation of the form

\[Ax^2+By^2+Cz^2+Dxy+Exz+Fyz+Gx+Hy+Jz+Yard=0. \nonumber\]

To sketch the graph of a quadric surface, start by sketching the traces to understand the framework of the surface.
Of import quadric surfaces are summarized in Figures $\PageIndex{13}$ and $\PageIndex{xiv}$.

Glossary

cylinder: a set of lines parallel to a given line passing through a given bend

ellipsoid: a iii-dimensional surface described past an equation of the form $ \dfrac{x^2}{a^2}+\dfrac{y^2}{b^2}+\dfrac{z^2}{c^2}=1$; all traces of this surface are ellipses

elliptic cone: a three-dimensional surface described by an equation of the grade $ \dfrac{x^2}{a^2}+\dfrac{y^two}{b^2}−\dfrac{z^2}{c^2}=0$; traces of this surface include ellipses and intersecting lines

elliptic paraboloid: a three-dimensional surface described by an equation of the class $ z=\dfrac{ten^2}{a^two}+\dfrac{y^2}{b^2}$; traces of this surface include ellipses and parabolas

hyperboloid of one sheet: a iii-dimensional surface described past an equation of the course $ \dfrac{x^2}{a^2}+\dfrac{y^2}{b^2}−\dfrac{z^2}{c^2}=one;$ traces of this surface include ellipses and hyperbolas

hyperboloid of ii sheets: a 3-dimensional surface described by an equation of the form $ \dfrac{z^2}{c^ii}−\dfrac{x^two}{a^2}−\dfrac{y^2}{b^2}=ane$; traces of this surface include ellipses and hyperbolas

quadric surfaces: surfaces in iii dimensions having the property that the traces of the surface are conic sections (ellipses, hyperbolas, and parabolas)

rulings: parallel lines that make up a cylindrical surface

trace: the intersection of a three-dimensional surface with a coordinate plane

Contributors and Attributions

Gilbert Strang (MIT) and Edwin "Jed" Herman (Harvey Mudd) with many contributing authors. This content by OpenStax is licensed with a CC-Past-SA-NC iv.0 license. Download for gratuitous at http://cnx.org.

yanggial1947.blogspot.com

Source: https://math.libretexts.org/Bookshelves/Calculus/Book%3A_Calculus_(OpenStax)/12%3A_Vectors_in_Space/12.6%3A_Quadric_Surfaces

drawing traces to sketch 3d object

12.6: Quadric Surfaces

Identifying Cylinders

Quadric Surfaces

Central Concepts

Glossary

Contributors and Attributions

0 Response to "drawing traces to sketch 3d object"

Post a Comment

Iklan Atas Artikel

Iklan Tengah Artikel 1

Iklan Tengah Artikel 2

Iklan Bawah Artikel